|
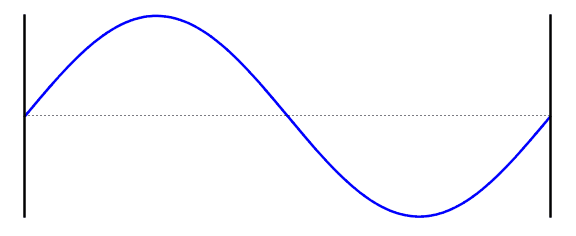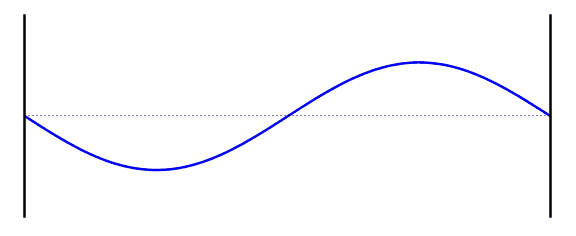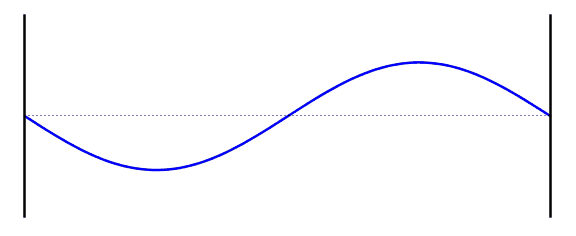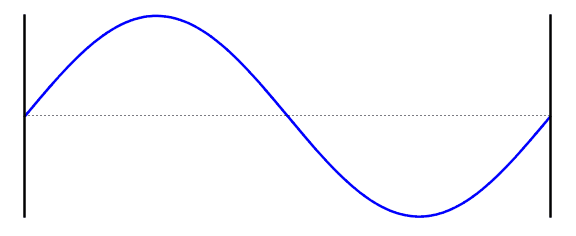
When a standing wave forms on a string, there is always a node at each end. The fundamental mode of vibration is simplest standing wave on a string, with a single antinode at the center and a node at each end. This is also known as the first harmonic. For the first harmonic, the length of the string is equal to one half of a wavelength of the standing wave, because the distance from one node to the next nearest node is one half a wavelength. The frequency of the first harmonic is called the fundamental frequency (f0) | |
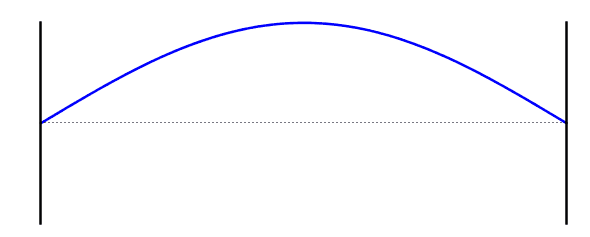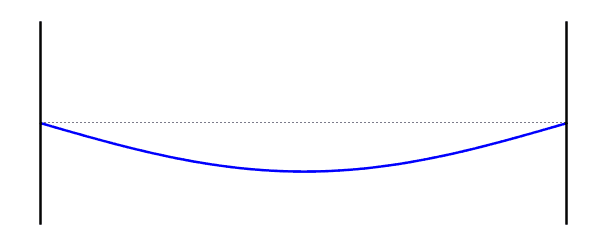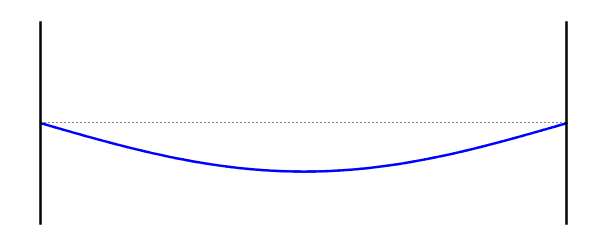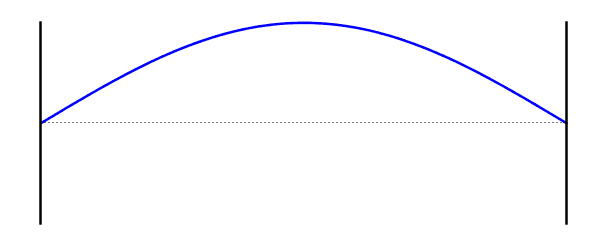 Fundamental Frequency (First Harmonic) |
f = f0 L = λ/2 λ = 2L |
| There are many other modes of vibration for a string fixed at each end. Each mode is called a harmonic. At the 2nd harmonic the standing wave consists of two segments. The wavelength is half the wavelength of the fundamental and therefore the frequency is twice that of the fundamental. | |
 2nd Harmonic |
f = 2f0 L = λ λ = L |
| At the 3rd harmonic the standing wave consists of three segments. The wavelength is one third the wavelength of the fundamental and therefore the frequency is three times that of the fundamental. | |
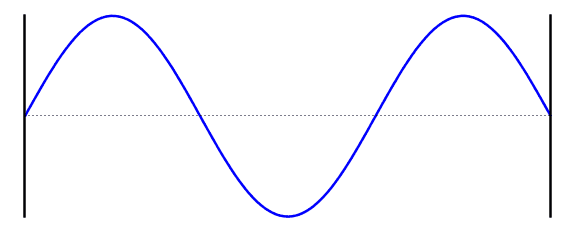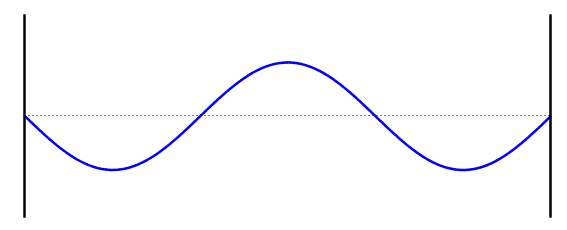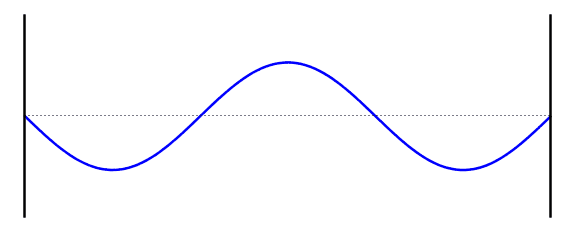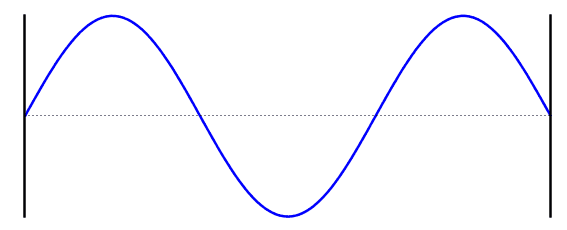 3rd Harmonic |
f = 3f0 L = 3λ/2 λ = 2L/3 |